Wireless Channel Generation for Multiple Carrier Frequencies

  • In this tutorial, we will analyze the performance of channel Model at multiple carrier frequencies under the propagation scenario Urban Macro or “UMa” for a Hexagonal Base Station (BS) Layout.

  • For a given number of BSs and UEs we generate multi-frequency cluster level channel coefficients corresponding to every link being simulated.

  • We first import the necessary libraries then followed by creating objects of classes AntennaArrays, NodeMobility, and SimulationLayout respectively.

The content of the tutorial is as follows:

Table of Contents

Import Libraries

Python Libraries

[1]:

import os
os.environ["CUDA_VISIBLE_DEVICES"] = "-1"
os.environ['TF_CPP_MIN_LOG_LEVEL'] = '3'

# %matplotlib widget
import matplotlib.pyplot as plt
import matplotlib as mpl
import numpy      as np

5G Toolkit Libraries

[2]:

# importing necessary modules for simulating channel model
# import sys
# sys.path.append("../../../")

from toolkit5G.ChannelModels import NodeMobility
from toolkit5G.ChannelModels import AntennaArrays
from toolkit5G.ChannelModels import SimulationLayout
from toolkit5G.ChannelModels import ParameterGenerator
from toolkit5G.ChannelModels import ChannelGenerator

Simulation Parameters

The simulation parameters are defined as follows * propTerrain defines propagation scenario or terrain for BS-UE, UE-UE, BS-BS links * carrierFrequency defines array of carrier frequencies in GHz * nBSs defines number of Base Stations (BSs) * nUEs defines number of User Equipments (UEs) * nSnapShots defines number of SnapShots

[3]:

# Simulation Parameters
propTerrain      = "UMa"                          # Propagation Scenario or Terrain for BS-UE links
carrierFrequency = np.array([3*10**9, 28*10**9])  # Array of two carrier frequencies in Hz
nBSs             = 21                             # number of BSs
nUEs             = 50                             # number of UEs
nSnapShots       = 10                             # number of SnapShots

Generate Antenna Array

Antenna Arrays for UEs

The following steps describe the procedure to simulate AntennaArrays Objects at a single carrier frequency both at Tx and Rx side:

[4]:

# Antenna Array at UE side
# assuming antenna element type to be "OMNI"
# with 2 panel and 2 single polarized antenna element per panel.
numCarriers = carrierFrequency.shape[0]
ueAntArray  = np.empty(numCarriers, dtype=object)
for i in range(carrierFrequency.size):
    ueAntArray[i] = AntennaArrays(antennaType     = "OMNI",
                                  centerFrequency = carrierFrequency[i],
                                  arrayStructure  = np.array([1,1,2,2,1]))
    ueAntArray[i]()
# Radiation Pattern of Rx antenna element
ueAntArray[0].displayAntennaRadiationPattern()
../../../_images/api_Tutorials_Tutorial5_6.Generate_UMa_Channel_for_Multiple_Frequencies_8_0.png 
[4]:

(<Figure size 960x480 with 1 Axes>, <Axes3D: >)

[20]:

ueAntArray[0].displayArray("2D", markerSize = 288)
../../../_images/api_Tutorials_Tutorial5_6.Generate_UMa_Channel_for_Multiple_Frequencies_9_0.png 
[20]:

(<Figure size 640x480 with 1 Axes>, <Axes: >)

Antenna Arrays for BS

[6]:

# Antenna Array at BS side
# assuming antenna element type to be "3GPP_38.901", a parabolic antenna
# with 4 panel and 4 single polarized antenna element per panel.
numCarriers    = carrierFrequency.shape[0]
bsAntArray     = np.empty(numCarriers, dtype=object)
for i in range(carrierFrequency.size):
    bsAntArray[i] = AntennaArrays(antennaType     = "3GPP_38.901",
                                  centerFrequency = carrierFrequency[i],
                                  arrayStructure  = np.array([1,1,4,4,1]))
    bsAntArray[i]()
# Radiation Pattern of Tx antenna element
bsAntArray[0].displayAntennaRadiationPattern()
../../../_images/api_Tutorials_Tutorial5_6.Generate_UMa_Channel_for_Multiple_Frequencies_11_0.png 
[6]:

(<Figure size 960x480 with 1 Axes>, <Axes3D: >)

[19]:

bsAntArray[0].displayArray("2D", markerSize = 288)
../../../_images/api_Tutorials_Tutorial5_6.Generate_UMa_Channel_for_Multiple_Frequencies_12_0.png 
[19]:

(<Figure size 640x480 with 1 Axes>, <Axes: >)

Node Mobility

This subsection provides the following steps to simulate the mobility of each node

[8]:

# NodeMobility parameters
# assuming that all the BSs are static and all the UEs are mobile.
interval = 10*0.5*10**-3/nSnapShots
timeInst = np.arange(nSnapShots, dtype=np.float32)*interval  # time values at each snapshot.
UEroute  = NodeMobility("randomWalk", nUEs, timeInst, 0, 10)
UEroute.displayRoute()
../../../_images/api_Tutorials_Tutorial5_6.Generate_UMa_Channel_for_Multiple_Frequencies_15_0.png 
[8]:

(<Figure size 640x480 with 1 Axes>, <Axes: >)

Generate Simulation Layout

We define the simulation topology parametes:

  • ISD: Inter Site Distance

  • minDist: Minimum distance between transmitter and receiver.

  • bsHt: BS heights

  • ueHt: UE heights

  • topology: Simulation Topology

  • nSectorsPerSite: Number of Sectors Per Site

Furthermore, users can access and update following parameters as per their requirements for channel using the handle simLayoutObj.x where x is:

  • The following parameters can be accessed or updated immendiately after object creation

    • UEtracks

    • UELocations

    • ueOrientation

    • UEvelocityVector

    • BStracks

    • BSLocations

    • bsOrientation

    • BSvelocityVector

  • The following parameters can be accessed or updated after calling the object

    • linkStateVec

[9]:

# Layout Parameters
isd                  = 500         # inter site distance
minDist              = 35          # min distance between each UE and BS
ueHt                 = 1.5         # UE height
bsHt                 = 25          # BS height
bslayoutType         = "Hexagonal" # BS layout type
ueDropType           = "Hexagonal" # UE drop type
nSectorsPerSite      = 3           # number of sectors per site

# simulation layout object
simLayoutObj = SimulationLayout(numOfBS = nBSs,
                                numOfUE = nUEs,
                                heightOfBS = bsHt,
                                heightOfUE = ueHt,
                                UEheightDistribution="equal",
                                UEdistibution="equal",
                                ISD = isd,
                                layoutType = bslayoutType,
                                ueDropMethod = ueDropType,
                                numOfSectorsPerSite = nSectorsPerSite,
                                ueRoute = UEroute)

simLayoutObj(terrain = propTerrain,
             carrierFreq = carrierFrequency,
             ueAntennaArray = ueAntArray,
             bsAntennaArray = bsAntArray)

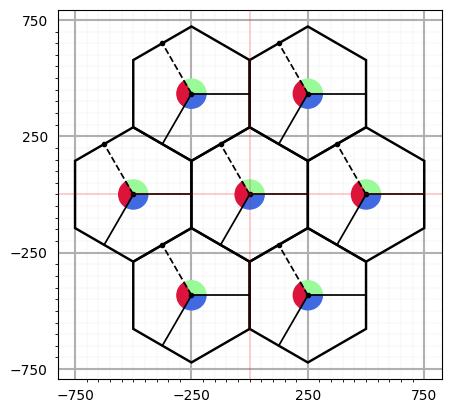
# displaying the topology of simulation layout
fig, ax = simLayoutObj.display2DTopology()
ax.set_xlabel("x-coordinates (m)")
ax.set_ylabel("y-coordinates (m)")
ax.set_title("Simulation Topology")
ax.legend()
../../../_images/api_Tutorials_Tutorial5_6.Generate_UMa_Channel_for_Multiple_Frequencies_17_0.png 
[9]:

<matplotlib.legend.Legend at 0x7f4c0d32b050>

Generate Channel Parameters

  • This subsection provides the steps to obtain all the cluster level channel parameters, which includes both Large Scale Parameters (LSPs) and Small Scale Parameters (SSPs).

  • LSPs includes Path Loss (PL), Delay Spread (DS) and Angular Spreads both in Azimuth and Zenith directions, and cluster powers (Pn) comes under SSPs.

  • LSPs/SSPs: paramGenObj.x where x is

    • linkStateVec

    • delaySpread

    • phiAoA_LoS, phiAoA_mn, phiAoA_spread

    • thetaAoA_LoS, thetaAoA_mn, thetaAoA_spread

    • phiAoD_LoS, phiAoD_mn, phiAoD_spread

    • thetaAoD_LoS, thetaAoD_mn, thetaAoD_spread

    • xpr

    • pathloss, pathDelay, pathPower

    • shadowFading

[10]:

# channel parameters
paramGenObj = simLayoutObj.getParameterGenerator()

Generate Channel Coefficients

Cluster level channel coefficients can be simulated using the following code snippet.

  • channel.coefficients with shape: (number of carrier frequencies, number of snapshots, number of BSs, number of UEs, numCluster/numPaths, number of Rx antennas, number of Tx antennas)

  • channel.delays with shape: (number of carrier frequencies, number of snapshots, number of BSs, number of UEs, numCluster/numPaths)

[11]:

channel = paramGenObj.getChannel(applyPathLoss = True)

Generate OFDM Channel

  • Shape of OFDM Channel:

    • Hf is of shape : (number of carrier frequencies, number of snapshots, number of BSs, number of UEs, fftsize, number of Rx antennas, number of Tx antennas)

[12]:

fftsize           = 512
subcarrierSpacing = 15*10**3
Hf = channel.ofdm(subcarrierSpacing, fftsize, simLayoutObj.carrierFrequency)
# Hf.shape: (numCarrierFrequencies, numSnapShots, numBSs, numUEs, Nfft, numRxAntennas, numTxAntennas)

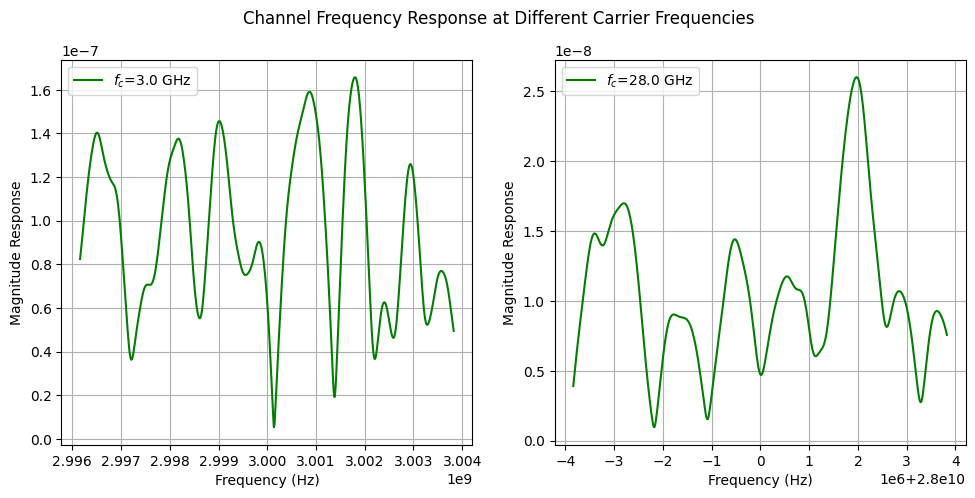
Frequency Domain : Magnitude Response Plot

  • The frequency domain magnitude plots (frequency responses) helps demonstate the order of frequency selectivity

    • Frequency selectivity is low for LOS Channel

    • frequency selectivity is high for NLOS Channels

  • Wireless channel at high frequency

    • has higher path-loss

    • less frequency selective (due to lower delay spread and weak distance paths)

[13]:

scaleFig = 1.5
fig, ax = plt.subplots(1,2,figsize=(17.5/scaleFig,7.5/scaleFig))
i = 0
ax[0].plot(np.arange(-channel.fftSize/2, channel.fftSize/2)*channel.subCarrierSpacing + channel.fc[0],
           np.abs(Hf[0,0,0,i,:,0,0]), "g", label = "$f_c$="+str(channel.fc[0]/10**9)+" GHz")

ax[1].plot(np.arange(-channel.fftSize/2, channel.fftSize/2)*channel.subCarrierSpacing + channel.fc[1],
           np.abs(Hf[1,0,0,i,:,0,0]), "g", label = "$f_c$="+str(channel.fc[1]/10**9)+" GHz")

ax[0].legend()
ax[0].set_xlabel("Frequency (Hz)")
ax[0].set_ylabel("Magnitude Response")
ax[0].grid()
ax[1].legend()
ax[1].set_xlabel("Frequency (Hz)")
ax[1].set_ylabel("Magnitude Response")
ax[1].grid()
fig.suptitle("Channel Frequency Response at Different Carrier Frequencies")

# plt.show()

[13]:

Text(0.5, 0.98, 'Channel Frequency Response at Different Carrier Frequencies')
../../../_images/api_Tutorials_Tutorial5_6.Generate_UMa_Channel_for_Multiple_Frequencies_25_1.png

Time Domain Channel response

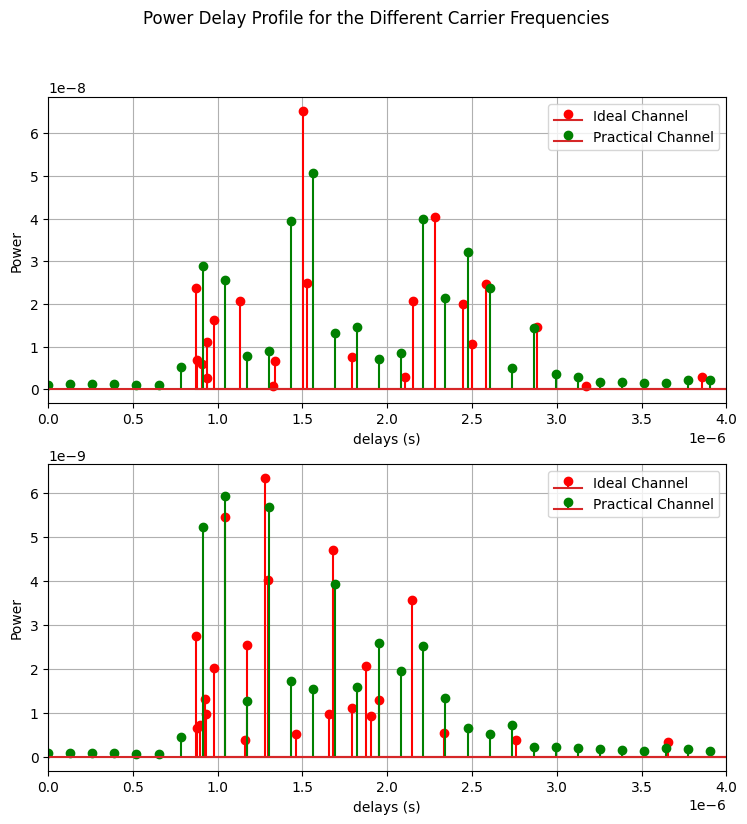
  • Practical wireless channel are bandlimited which results in:

    • impulses widening:

      • higher for lower frequency channels

    • time spread

These effects can be observed in following plots.

[14]:

ht = np.fft.ifft(Hf, fftsize, axis = -3)

[15]:

scaleFig = 2
fig, ax = plt.subplots(2,1,figsize=(17.5/scaleFig,17.5/scaleFig))
i = 0
ax[0].stem(channel.delays[0,0,0,i], np.abs(channel.coefficients[0,0,0,i,:,0,0]), "r", label = "Ideal Channel")
ax[0].stem(np.arange(fftsize)/(fftsize*channel.subCarrierSpacing), np.abs(ht[0,0,0,i,:,0,0]), "g", label = "Practical Channel")
ax[0].legend()
ax[0].set_xlim([0, 0.4*10**-5])
ax[0].set_xlabel("delays (s)")
ax[0].set_ylabel("Power")
ax[0].grid()

ax[1].stem(channel.delays[1,0,0,i], np.abs(channel.coefficients[1,0,0,i,:,0,0]), "r", label = "Ideal Channel")
ax[1].stem(np.arange(fftsize)/(fftsize*channel.subCarrierSpacing), np.abs(ht[1,0,0,i,:,0,0]), "g", label = "Practical Channel")
ax[1].legend()
ax[1].set_xlim([0, 0.4*10**-5])
ax[1].set_xlabel("delays (s)")
ax[1].set_ylabel("Power")
ax[1].grid()

fig.suptitle("Power Delay Profile for the Different Carrier Frequencies")
plt.show()
../../../_images/api_Tutorials_Tutorial5_6.Generate_UMa_Channel_for_Multiple_Frequencies_28_0.png 
[ ]: